Introduction to Hexagonal Geodesic Domes

However, this is not how nature builds apparatus such as the eyes of flies:

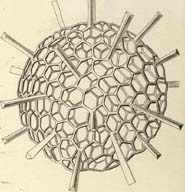
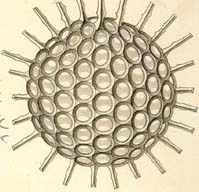
Also, it's not what Haekel's drawings of radiolaria showed:

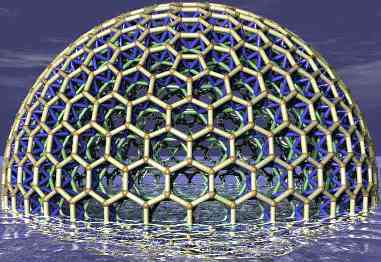
One reason for that is that - while triangles are very strong - sheets constructed from them are very wasteful of structural material.

The most efficient structure to use to tesselate into a two-dimensional spaces is usually hexagonal.

Soccer balls illustrate the layout of hexagonal domes. The resulting polyhedra are known as "duals" of the polyhedra of conventional geodesic domes.

However, such domes are not very common.

One possible reason for that is that the most basic hexagonal dome structure is not stable.

The following photograph shows such a structure suspended from one node - to illustrate the nature of the problem:

Hexagonal geodesic domes

In fact, a number of large hexagonal domes have been built:

The Montreal dome:

This dome illustrates the simplest dual-layer tetrahedral-truss design.

The Eden Project domes:

The Eden Project domes utilise an unusual tetrahedral-truss - which requires relatively little additional material and results in a remarkably thin dome.

This configuration is also reasonably stable - though it has some stability issues when compared to structures which use more material - such as the Montreal dome.

There are many more physical hexagonal domes at the top of this site's links section.

Two roles

• Large, strong struts form the hexagonal shell:
  
  • These struts provide the primary weight-bearing support for the dome.

• Truss struts are narrower and lighter:
  
  • These struts provide stability for the dome.

Two aproaches

There are essentially two main sorts of approach:

• Use supporting struts;

• Use tension elements.

• Using supporting struts

  Most of the existing hexagonal geodesic domes use supporting struts.

• Using tensegrity

  Conventionally, structures containing tension elements which are necessary to their structural integrity are known as "tensegrities".

  Using tensegrity has a number of attractions - in particular the resulting structures can be stable and strong - but are also light, cheap and resiliant.

  A simple tensegrity is pictured here:

  
  

  Domes have been constructed in the past using the principles of tensegrity. However they have tended to offer an external surface which consists of tension elements.

  Having external tension elements may be acceptable when throwing a tarpaulin over the resulting dome - but presents some challenges when more conventional roofing approached are applied.

  This site offers a new aproach to stabilising domes based on an external structural shell consisting of a hexagon-pentagon tesselation - using the principles of tensegrity.

  The following photograph shows a chopstick dodecahedron reinforced using a single layer of string:

  
  

  The structure deforms negligibly under its own weight.

  
  

  Also, it easily supports its own weight.

  The idea of using tension elements to replace stabilising struts - takes the natural split into two types of structural material often found in hexagonal domes to an extreme.

  The stabilising components do not need to support compressive forces to do their job effectively. Use of tension elements can have cost and weight advantages - since breaking strains are often dramatically higher than buckling stresses in materials of equivalent weight or cost.

For more details about this, see the simulation page.

Advantages

Converting a conventional triangular geodesic dome into a hexagonal dome containing the same volume constits of eliminating two thirds of the struts - and about a third of the hubs.

Most of the struts in a conventional geodesic dome are pretty redundant - and are not really necessary for the structure to retain its structural integrity.

Also, triangular regions present some problems when roofing - due to problems in the corners of the triangles. These are stressed regions - and are also points of reduced strength. Replacing the triangles with hexagons neatly solves this problem.

Hexagonal domes also distribute strength more evenly over their surfaces - strength of triangular domes is concentrated more at their hubs. Hexagonal domes do not have such heavy concentrations of structural material at particular points.

Big domes

The answer to that is simple: when building large domes.

Small domes do not have significant difficulties supporting their own weight. Component costs are also very significant (compared to labour costs).

However weight becomes increasing significant as the size of the dome increases - until it is a heavily dominant factor.

Because domes built using strong weight-bearing components and lighter stabilising components can be made significantly lighter than conventional geodesic domes, they can be made proportionally larger.

The principle of using the minimum quantity of weight- bearing materials (and using more lightweight materials to provide stability) becomes increasingly important as the size of the dome increases.

With a small dome hexagonal geometry and multiple layers are a complication - in very large domes they are practically a necessity.

Thickness

Many such hexagonal domes have been constructed.

In theory more thickness than is provided by two layers may be needed for very large domes. The most obvious approach under such circumstances would be to add more layers.

Domes with more than one hexagonal layer have been constructed - see the sections devoted to the ASM dome and the Climatron in our links section.

The hexagonal layers would be weight-bearing, the triangular layers stabilising - with everything connected by tetrahedra.