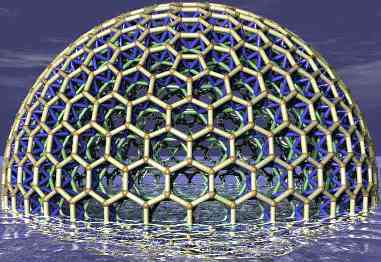
HexDome
Hexagonal Geodesic Domes
Pentagonal prism tensegrity
Here's a "floating strut" tensegrity.

Pentagonal prism
|
Note that the struts do not touch each other.
It is an unusual structure - since it contains no triangles.
Triangulation
Some people seem to think that structures are necessarily dependent on
the presence of triangles.
They sometimes cite Buckminster Fuller on the issue:
"Only the triangle produces structure and structure means only triangle; and visa versa"
- Synergetics, 608.05.
"By structure we mean omnitriangulated. The triangle is the only structure."
- Synergetics, 610.02.
"If we want to have a structure, we have to have triangles."
- Synergetics, 610.12.
However, no coherent explanation of why this might be so appears to have been
offered. At one point he says:
"we have learned experimentally that only triangles are stable"
- Synergetics, 401.03.
Structures like this one appear to represent experimental counter-examples to
the notion that stability depends on the presence of triangles.
The apparent stability of this totally triangle-free configuration surely casts
serious doubt on the dogma that triangles necessarily form the basis of all
stable structures.
Synergy
That this structure appears to be stable makes it a good example of structural
synergy.
It's also of interest since each strut is attached at each
end to three cables - the minimum possible for a
"floating-strut" tensegrity.
This is the simplest model with these properties that has
been found - though it may be possible for a similar
four-strut system to be constructed.

Pentagonal prism
|
Links
Pandia Raj Ramar's pentagonal model
Adrian Rossitor's hexagonal model
Bob Burkhardt's hexagonal model
T-Chime pentagonal model - probably a different cable configuration
Tim Tyler |
Contact |
http://hexdome.com/
|