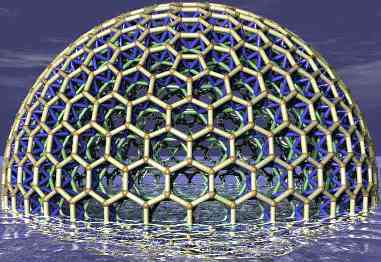
HexDome
Hexagonal Geodesic Domes
Goldberg greenhouse conjectures
Joe Clinton's
[Equal Central Angle Conjecture]
suggests it might be possible to
deform Goldberg polyhedra in such a
way that their vertices remain on
a sphere, while all their sides come
to have equal lengths.
It occurs to me that there are
some other related equality
conjectures for Goldberg structures.
In particular it might be possible
to deform a Goldberg polyhedron in
such a way that their vertices remain
on a sphere while all the areas
of their faces became equal.
A similar equality conjecture is based
on the observation that the required
strength of the laminar material required
to cover a region is generally a
function of the largest distance it has to
span:
It may be possible to deform a Goldberg
polyhedron in such a way that all their faces
retain co-planar vertices, while the distances
of the points near the middle of each face which
are furthest from any edge all become equal.
Defining "area" of a face which may not have
co-planar vertices - and decdiding which
point of such a face is furthest from an edge
are issues not addressed here - except by noting
that these are tricky issues - but that there
are solutions to them.
Lastly, I note that it may be possible to
create three new conjectures (equal angle, equal
area, equal span) by dropping the constraint that
the points must all remain on the surface of a
sphere - and adding the constraint that the vertices
of each face remain co-planar.
The equal-angle conjecture is associated
with the problem of minimising the number
of cuts during construction.
These conjectures are motivated by considerations
of structures which could be covered by panes of
glass - where co-planar faces is critical - and
where having variations in the maximum unbroken
span might result in variations in the strength
of the panes, for a given thickness of material.
Tim Tyler |
Contact |
http://hexdome.com/
|